Express The Given Hindu Arabic Numeral In Expanded Form - ( 9 × 1 0 1 ) + ( 4 × 1 ) \left(9 \times 10^{1}\right)+(4 \times 1) ( 9 × 1 0 1 ) + ( 4 × 1 ) solution

Express The Given Hindu Arabic Numeral In Expanded Form - Web you'll get a detailed solution from a subject matter expert that helps you learn core concepts. We start by showing all powers of 10, starting with the highest exponent given. 7647 7647 = (use the multiplication symbol in the math palette as needed. ( 7 × 1 0 1 ) + ( 3 × 1 ) \left(7 \times 10^{1}\right)+(3 \times 1) ( 7 × 1 0 1 ) + ( 3 × 1 ) solution (5x103) + (0x102) + (0x104) + (8x1) (5x103) + (0x102) +.

Web the evolution of a system. This problem has been solved!. (7 × 103) + (5 × 101) + (4 × 1). Do not perform the calculation.). ( 9 × 1 0 1 ) + ( 4 × 1 ) \left(9 \times 10^{1}\right)+(4 \times 1) ( 9 × 1 0 1 ) + ( 4 × 1 ) solution V this problem has been solved! 5,000 + 300 + 20 + 5 = 5,325 expanded factors form:

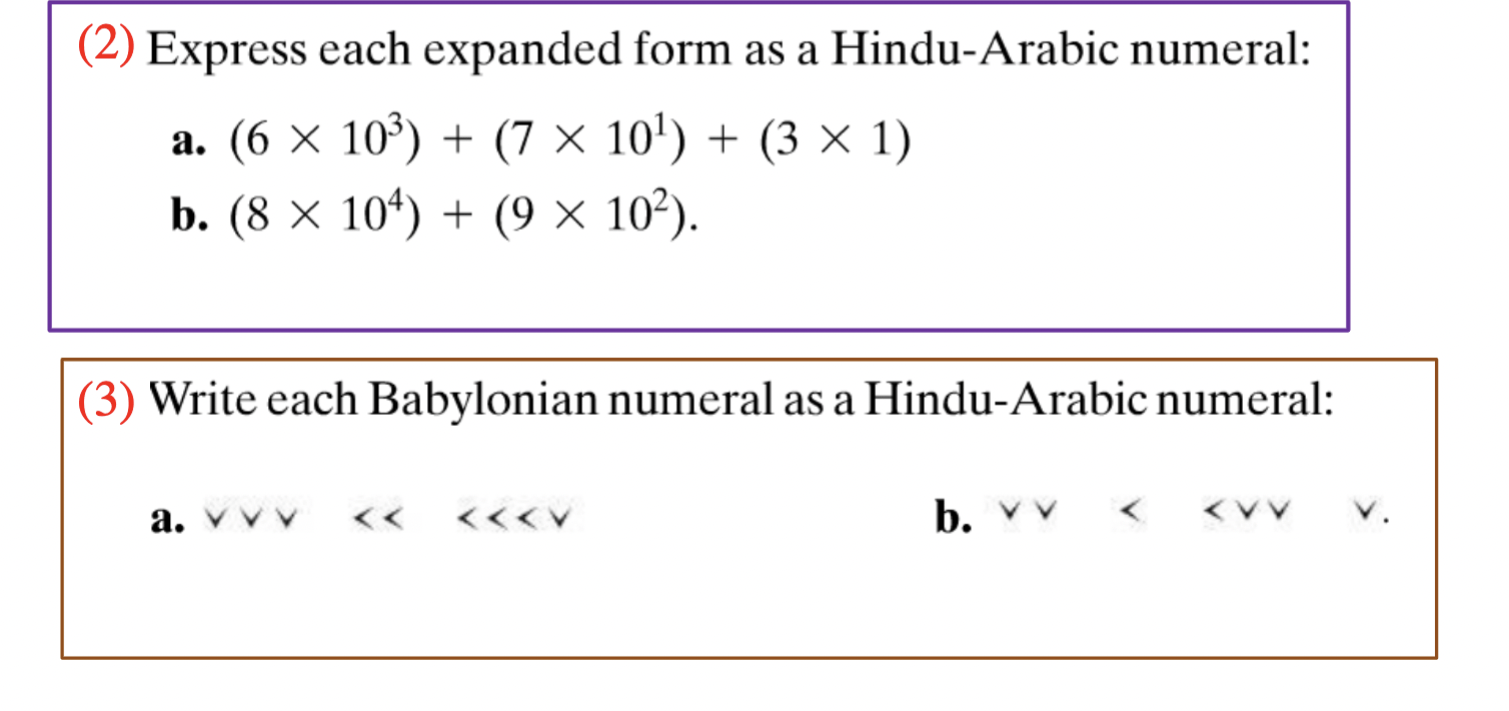
Solved (2) Express each expanded form as a HinduArabic

Solved (2) Express each expanded form as a HinduArabic

(7 × 103) + (5 × 101) + (4 × 1). You'll get a detailed solution from a subject matter expert that. Parenthesis four times ten to the fourth power close parenthesis, plus parenthesis seven. 5,000 + 300 + 20 + 5 = 5,325 expanded factors form: (3 × 1 0 2) + (8 ×.

The Hindu—Arabic Number System and Roman Numerals (2023)

The Hindu—Arabic Number System and Roman Numerals (2023)

7647 7647 = (use the multiplication symbol in the math palette as needed. (7 × 103) + (5 × 101) + (4 × 1). You'll get a detailed solution from a subject matter expert that. Do not perform the calculation.). (5x103) + (0x102) + (0x104) + (8x1) (5x103) + (0x102) +. The modern system of.

Writing HinduArabic Numerals in Expanded Form

Writing HinduArabic Numerals in Expanded Form

The modern system of counting and computing isn’t. Web the evolution of a system. Parenthesis four times ten to the fourth power close parenthesis, plus parenthesis seven. Do not perform the calculation.). ( 7 × 1 0 1 ) + ( 3 × 1 ) \left(7 \times 10^{1}\right)+(3 \times 1) ( 7 × 1 0.

Answered Express each expanded form as a… bartleby

Answered Express each expanded form as a… bartleby

( 9 × 1 0 1 ) + ( 4 × 1 ) \left(9 \times 10^{1}\right)+(4 \times 1) ( 9 × 1 0 1 ) + ( 4 × 1 ) solution V this problem has been solved! The modern system of counting and computing isn’t. You'll get a detailed solution from a subject matter.

Writing HinduArabic Numerals in Expanded Form

Writing HinduArabic Numerals in Expanded Form

Web you'll get a detailed solution from a subject matter expert that helps you learn core concepts. (1× 102)+ (5× 101)+ (7× 1) 13 35 130 157. This problem has been solved!. (7 × 103) + (5 × 101) + (4 × 1). Parenthesis four times ten to the fourth power close parenthesis, plus parenthesis.

[Solved] Express the given expanded numeral as a HinduArabic numeral

[Solved] Express the given expanded numeral as a HinduArabic numeral

Do not perform the calculation.). 7647 7647 = (use the multiplication symbol in the math palette as needed. Web the evolution of a system. (5 × 1,000) + (3 × 100) + (2 × 10) + (5 × 1) = 5,325 expanded exponential form: ( 7 × 1 0 1 ) + ( 3 ×.

[Solved] Express the given HinduArabic numeral in expanded form. 907 O

[Solved] Express the given HinduArabic numeral in expanded form. 907 O

Web the evolution of a system. Web you'll get a detailed solution from a subject matter expert that helps you learn core concepts. 100% (1 rating) transcribed image text: V this problem has been solved! The modern system of counting and computing isn’t. (5 × 103) + (3 × 102) + (2 × 101) +.

[ANSWERED] Use the table to write the given Hindu Arabic numera

[ANSWERED] Use the table to write the given Hindu Arabic numera

Web you'll get a detailed solution from a subject matter expert that helps you learn core concepts. Web the evolution of a system. (7 × 103) + (5 × 101) + (4 × 1). (5 × 1,000) + (3 × 100) + (2 × 10) + (5 × 1) = 5,325 expanded exponential form: (5.

Writing HinduArabic Numerals in Expanded Form

Writing HinduArabic Numerals in Expanded Form

(1× 102)+ (5× 101)+ (7× 1) 13 35 130 157. ( 7 × 1 0 1 ) + ( 3 × 1 ) \left(7 \times 10^{1}\right)+(3 \times 1) ( 7 × 1 0 1 ) + ( 3 × 1 ) solution This problem has been solved!. (5x103) + (0x102) + (0x104) + (8x1) (5x103).

PPT 4.1 PowerPoint Presentation, free download ID5936567

PPT 4.1 PowerPoint Presentation, free download ID5936567

Parenthesis four times ten to the fourth power close parenthesis, plus parenthesis seven. V this problem has been solved! The modern system of counting and computing isn’t. 100% (1 rating) transcribed image text: Do not perform the calculation.). We start by showing all powers of 10, starting with the highest exponent given. (5 × 1,000).

Express The Given Hindu Arabic Numeral In Expanded Form We start by showing all powers of 10, starting with the highest exponent given. Web you'll get a detailed solution from a subject matter expert that helps you learn core concepts. ( 7 × 1 0 1 ) + ( 3 × 1 ) \left(7 \times 10^{1}\right)+(3 \times 1) ( 7 × 1 0 1 ) + ( 3 × 1 ) solution V this problem has been solved! (5 × 1,000) + (3 × 100) + (2 × 10) + (5 × 1) = 5,325 expanded exponential form:

(5 × 103) + (3 × 102) + (2 × 101) + (5 × 100) = 5,325 Word Form:

We start by showing all powers of 10, starting with the highest exponent given. (5x103) + (0x102) + (0x104) + (8x1) (5x103) + (0x102) +. This problem has been solved!. The modern system of counting and computing isn’t.

(1× 102)+ (5× 101)+ (7× 1) 13 35 130 157.

Do not perform the calculation.). You'll get a detailed solution from a subject matter expert that. V this problem has been solved! (5 × 1,000) + (3 × 100) + (2 × 10) + (5 × 1) = 5,325 expanded exponential form:

7647 7647 = (Use The Multiplication Symbol In The Math Palette As Needed.

Parenthesis four times ten to the fourth power close parenthesis, plus parenthesis seven. 100% (1 rating) transcribed image text: ( 7 × 1 0 1 ) + ( 3 × 1 ) \left(7 \times 10^{1}\right)+(3 \times 1) ( 7 × 1 0 1 ) + ( 3 × 1 ) solution (3 × 1 0 2) + (8 × 1 0 1) + (5 × 1) \left(3 \times 10^{2}\right)+\left(8 \times 10^{1}\right)+(5 \times 1) (3 × 1 0 2) + (8 × 1 0.

( 9 × 1 0 1 ) + ( 4 × 1 ) \Left(9 \Times 10^{1}\Right)+(4 \Times 1) ( 9 × 1 0 1 ) + ( 4 × 1 ) Solution

32,714 32,714 = 1 (use the multiplication symbol in the math palette as needed. Web you'll get a detailed solution from a subject matter expert that helps you learn core concepts. Web the evolution of a system. 5,000 + 300 + 20 + 5 = 5,325 expanded factors form:

Express The Given Hindu Arabic Numeral In Expanded Form Related Post :